Geometric Progression Sum to Infinity
The number of rectangles in a square grid is given by the squared triangular numbers. And r should not be 0 because the sequence a00 is not geometric.
Geometric Progression GP.
. However if the set to which the terms and their finite sums belong has a notion of limit it is sometimes possible to assign a value to a series called the sum of the seriesThis value is the limit as n tends to infinity if the limit exists of the finite sums of. An arithmetic-geometric progression AGP is a progression in which each term can be represented as the product of the terms of an arithmetic progressions AP and a geometric progressions GP. Lesson 31 - Triangle Sum Theorem Proof Triangle Sum Theorem Proof.
Find the sum to infinity of each geometric sequence if it exists. Intuitively the sum of an infinite number of terms will be equal to infinity whether the common difference is positive negative or even equal to zero. In this section we will learn to find the sum of geometric series.
Lesson 4 - Geometric Shapes. For example the series is geometric because each successive term can be obtained by multiplying the previous term by In general a geometric series is written as where is the coefficient of each term and is the common ratio. So what happens when n goes to infinity.
So far we have talked about geometric sequences or geometric progressions which are collections of numbers. The first ten powers of 2 for non-negative values. The sum of the infinite GP formula is given as S n a1r where r.
Each successive term is obtained in a geometric progression by multiplying the common ratio to its preceding term. S n n22a n 1 d This is. Then the member terms grow towards negative infinity.
Nth Term of a GP. It is very useful while calculating the Geometric mean of the entire. The formula for the n th term of an AP.
The formula for the nth term of a geometric progression whose first term is a and common ratio is r is a n ar n1. 64 16 4 1. The list of formulas related to GP is given below which will help in solving different types of problems.
The sum of even numbers from 2 to infinity can be easily found using arithmetic progression as the set of even numbers is also an arithmetic progression with a fixed difference between any two consecutive terms. N th term for the GP. If a is the initial term and d is a common difference.
But be careful. That is the solution to the puzzle is given by the n th square pyramidal number. A n ar n-1.
The sum of the infinite series 1 2. Its geometric mean is zero and the harmonic mean is infinity. Lesson 14 - What is Infinity in Math.
The Product of all the numbers present in the geometric progression gives us the overall product. Geometric Sequences and Sums Sequence. Sum of the first n terms S n.
Pingala 300 BCE 200 BCE Among the scholars of the. In the example above this gives. So our infnite geometric series has a finite sum when the ratio is less than 1.
A geometric series is the sum of the numbers in a geometric progression. It is not the case for all types of sequences though. Since we know in a GP the common ratio between the successive terms is constant so we will consider a geometric series of finite terms to derive the formula to find the sum of Geometric Progression.
This is called the geometric progression formula of sum to infinity. How do we check whether a series is an arithmetic progression or not. Practice more questions.
Letting a be the first term here 2 n be the number of terms here 4 and r be the constant that each term is multiplied by to get the next term here 5 the sum is given by. Consider an AP consisting n terms. Find the infinite sum of the following infinite geometric series.
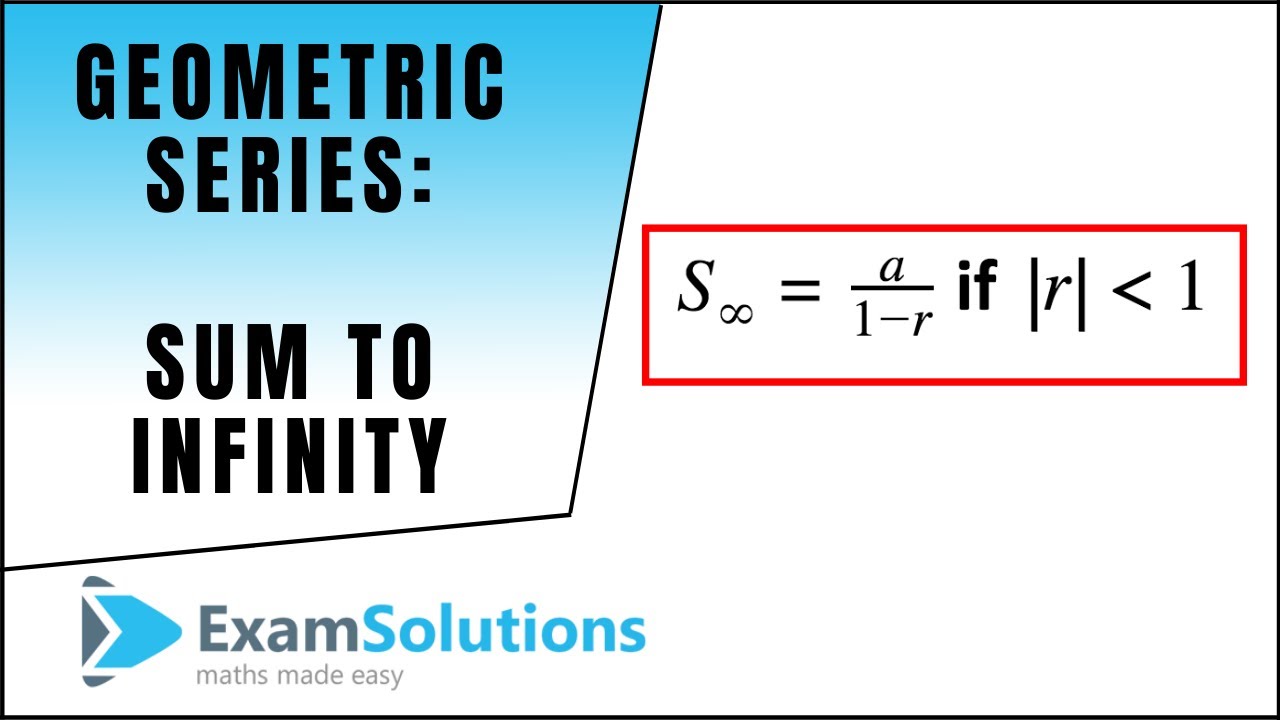
Here a is the first term and r is the common ratio. As with any infinite series the sum is defined to mean the limit of the partial sum of the first n terms as n approaches infinity. For Infinite Geometric Series.
Geometric Sum to Infinity. A power of two is a number of the form 2 n where n is an integer that is the result of exponentiation with number two as the base and integer n as the exponent. 8 terms of 3 3 3 3.
The general form of terms of a GP is a ar ar 2 ar 3 and so on. . 11X1 T10 03 arithmetic geometric means.
A Sequence is a set of things usually numbers that are in order. The formula works for any real numbers a and r except r 1. Sum to n Terms of a GP.
An important landmark of the Vedic period was the work of Sanskrit grammarian Pāṇini c. Problems based on Sum to n Terms of a GP. As n approaches infinity the term approaches 0 and so s n tends to 1.
By various arguments one can show that this finite sum is equal to. A geometric sequence also called a geometric progression GP. Must contain at least 4 different symbols.
6 to 30 characters long. If you pick another one for example a geometric sequence the sum to infinity might turn out to be a finite term. In mathematics a geometric series is the sum of an infinite number of terms that have a constant ratio between successive terms.
This series was used as a representation of many of Zenos. However there are really interesting results to be obtained when you try to sum the terms of a geometric sequenceWhen we have a finite geometric progression which has a limited number of terms the process here is as simple as finding the. Consider the GP a ar ar 2ar n-1.
N will tend to Infinity n Putting this in the generalized formula. View solution Sum to infinity of the series 3 2. The square pyramidal number also counts the number of acute triangles formed from the vertices of a -sided regular polygonFor instance an equilateral triangle contains only one acute triangle itself a regular.
If r 1 r 1 r 1 then the sum to infinity is given by. Whats the sum of the first 100 positive integers. A geometric progression is a sequence of numbers in which any two consecutive terms of the sequence have a common ratio.
The infinite sequence of additions implied by a series cannot be effectively carried on at least in a finite amount of time. Mean or average of the set of numbers which is computed by adding all the terms in the set of numbers and dividing the sum by a total number of terms. The formula for the arithmetic progression sum is explained below.
We can use this formula. Derivation of Sum of GP. Thats where limits come in.
ASCII characters only characters found on a standard US keyboard. His grammar includes early use of Boolean logic of the null operator and of context free grammars and includes a precursor of the BackusNaur form used in the description programming languages. Product of the Geometric series.
4 4 4 4 B. How about the first 1000. Thus the explicit formula is.
Grade 10 Science Module 1st Quarter Luwen Borigas. In a context where only integers are considered n is restricted to non-negative values so there are 1 2 and 2 multiplied by itself a certain number of times. The arithmetic and geometric progression Maija Liepa.
The formula to find the sum of even numbers can be derived using the formula of the sum of natural numbers such as S 1234567n. Geometric series Jhon Paul Lagumbay. The sum of the first n terms of the AP series.
The formula for the sum of n terms of AP. What happens when a functions output isnt calculable directly eg at infinity but we still need to understand its behavior.
Sum Of Infinite Gp Formula Sum Of Infinite Terms Of Gp
Proof Of The Geometric Series Formula Finite Infinite Youtube
Finding The Sum Of An Infinite Geometric Series Youtube
Geometrical Progression Sum Of Infinite Terms Derivation Youtube
Lesson Video Sum Of An Infinite Geometric Sequence Nagwa
Mathwords Infinite Geometric Series

0 Response to "Geometric Progression Sum to Infinity"
Post a Comment